How to Find the Factors of a Number

In this article, we’re going to explore a fascinating concept called factors. Have you ever wondered how numbers can be divided into smaller parts? Well, that’s exactly what we’ll be learning about!

Numbers are all around us, and they help us count, measure, and understand the world. But did you know that numbers can also be broken down into smaller numbers? These smaller numbers are called factors, and they play an important role in mathematics.

Think about sharing your toys with friends. Let’s say you have 12 toys, and you want to share them equally among your three best buddies. How many toys will each friend get? By finding the factors of 12, we can figure that out!

Factors are like the building blocks of numbers. They are the numbers that can divide evenly into a given number, without leaving any leftovers or remainders. When we find the factors of a number, we discover all the smaller numbers that can be multiplied together to make that number.

In our example of 12 toys, the factors are the numbers that can divide evenly into 12. By finding these factors, we’ll know how many toys each friend will receive.

So, get ready to dive into the world of factors! We’ll learn how to find the factors of a number, what factors are, and how they can help us solve problems. Let’s explore the fascinating world of numbers and factors together!

What is a Number?

Before we delve deeper into factors, let’s make sure we understand what numbers are. Numbers are like special symbols that represent quantities or amounts. They help us count, measure, and describe things in the world around us.

You encounter numbers every day. When you count your toys, the number of books on a shelf, or even the number of friends you have, you’re using numbers to express how many there are.

Numbers can be as small as 0 or as big as you can imagine! Some examples of numbers are 1, 2, 5, 10, 20, 100, and so on. They can be whole numbers like 7 or 23, or they can have decimals like 3.14 or 0.5. Numbers can even be negative, like -10 or -2.5.

We can perform different operations with numbers, such as adding them together, subtracting them, multiplying them, or dividing them. These operations help us solve mathematical problems and understand the relationships between numbers.

Now that we have a basic understanding of numbers, let’s move on to exploring factors. Factors are like the puzzle pieces that fit together to form a number. By finding the factors of a number, we can discover its building blocks and understand how it can be divided into smaller parts.

What are Factors of a Number?

What are Factors?

Factors are the special numbers that can divide evenly into a given number. When we find the factors of a number, we discover all the smaller numbers that can be multiplied together to make that number.

Let’s take a closer look at how factors work. Imagine we have the number 12. We want to find its factors, which are the numbers that can divide 12 without leaving any leftovers or remainders.

The factors of 12 are the numbers that, when multiplied together, give us the result of 12. Let’s try to find them:

1 x 12 = 12

2 x 6 = 12

3 x 4 = 12

The factors of 12 are 1, 2, 3, 4, 6, and 12. These numbers divide 12 evenly and can be multiplied together to make 12.

Factors are like the different ingredients that can be combined to create a recipe. For example, if we consider the number 12 as a cake, the factors would be the individual components that make up the cake, such as the flour, sugar, eggs, and so on. Without these factors, the cake wouldn’t be complete!

It’s important to note that every number has at least two factors: 1 and itself. In the case of 12, the factors 1 and 12 are present. Sometimes, a number can have many more factors, depending on its value.

Factors are incredibly useful in various mathematical concepts. They help us simplify fractions, find common denominators, and even determine if a number is prime (having only two factors: 1 and itself). We’ll explore these concepts in more detail later on.

Now that we understand what factors are, let’s learn how to find them and unlock the secrets hidden within numbers!

How to Find Factors of a Number

Finding factors is like solving a puzzle to discover the smaller numbers that can divide evenly into a given number. Let’s explore the step-by-step process of finding factors:

Step 1: Start with the number you want to find the factors of. Let’s use the number 12 as an example.
Step 2: Begin by dividing the number by the smallest possible factor, which is 1. When we divide 12 by 1, we get 12 as the result.
Step 3: Move on to the next number, which is 2. Divide 12 by 2. If the division gives us a whole number without any remainder, then 2 is a factor of 12. In this case, 12 divided by 2 is 6, which is a whole number.
Step 4: Continue the process by trying the next number, which is 3. Divide 12 by 3. If the division gives us a whole number without a remainder, then 3 is a factor of 12. In this case, 12 divided by 3 is 4, which is a whole number.
Step 5: Keep going and try the next number, which is 4. Divide 12 by 4. If the division gives us a whole number without any remainder, then 4 is a factor of 12. In this case, 12 divided by 4 is 3, which is a whole number.
Step 6: Continue trying the next numbers in order. The next number after 4 is 5, but we can stop here because 5 is greater than 12. Since there are no more numbers left to try, we have found all the factors of 12.

The factors of 12 are 1, 2, 3, 4, 6, and 12. These are the numbers that can divide evenly into 12, without leaving any leftovers or remainders.

By following this step-by-step process, you can find the factors of any number. Remember, factors are like the keys that unlock the secrets within numbers, revealing their building blocks and allowing us to understand their composition.

Now that you know how to find factors, let’s explore some helpful rules that can make the process even easier!

Divisibility Rules

When finding factors, it can be helpful to know some simple rules that make the process easier and faster. These rules are called divisibility rules and can help determine if a number is divisible by certain smaller numbers without performing the division.

Let’s explore some common divisibility rules:

Divisibility by 2: A number is divisible by 2 if its last digit is 0, 2, 4, 6, or 8. For example, the number 16 is divisible by 2 because its last digit is 6.
Divisibility by 3: A number is divisible by 3 if the sum of its digits is divisible by 3. For example, the number 12 has digits 1 and 2, and their sum is 3, which is divisible by 3.
Divisibility by 4: A number is divisible by 4 if the last two digits form a number that is divisible by 4. For example, the number 24 is divisible by 4 because the last two digits, 2 and 4, form the number 24, which is divisible by 4.
Divisibility by 5: A number is divisible by 5 if its last digit is 0 or 5. For example, the number 35 is divisible by 5 because its last digit is 5.
Divisibility by 6: A number is divisible by 6 if it is divisible by both 2 and 3. For example, the number 36 is divisible by 6 because it is divisible by 2 and 3.
Divisibility by 9: A number is divisible by 9 if the sum of its digits is divisible by 9. For example, the number 54 has digits 5 and 4, and their sum is 9, which is divisible by 9.

Remember, these rules are shortcuts that can save time when trying to determine if a number is divisible by another number. They are particularly useful when finding factors, as you can quickly eliminate numbers that don’t meet these rules, focusing on the ones that are more likely to be factors.

By applying these divisibility rules, you can make the process of finding factors even more efficient and enjoyable. Now, let’s put these rules into practice and find some factors of different numbers!

Finding Factors of a Number in Practice

Now that we understand how to find factors and have learned some handy divisibility rules, let’s put our knowledge into practice! We’ll work through a few examples together, finding the factors of different numbers step by step.

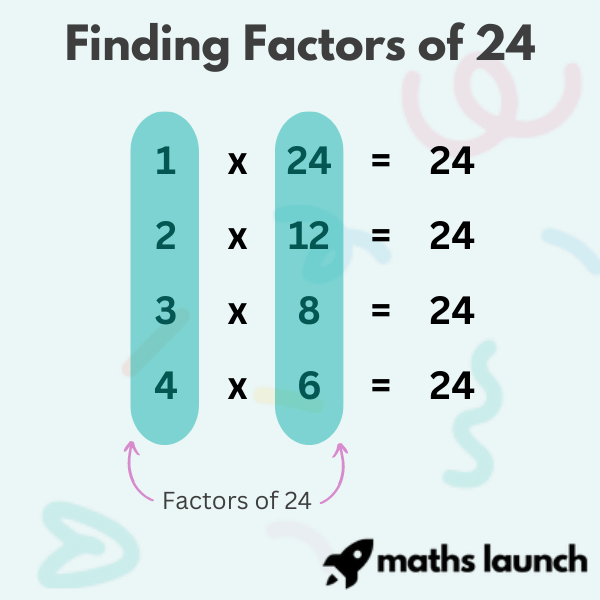
Example 1: Finding Factors of 24

Step 1: Start with the number 24.

Step 2: Divide 24 by 1. Since the result is 24, we have found the factor 1.

Step 3: Divide 24 by 2. The result is 12, so 2 is a factor.

Step 4: Divide 24 by 3. Since the result is not a whole number, 3 is not a factor.

Step 5: Divide 24 by 4. The result is 6, so 4 is a factor.

Step 6: Continue trying the next numbers. Divide 24 by 5, 6, 7, 8, and 9. None of these numbers divide 24 evenly.

Step 7: Finally, divide 24 by 10. The result is not a whole number, so 10 is not a factor.

Step 8: We can stop here since we have exhausted all possible numbers. The factors of 24 are 1, 2, 3, 4, 6, 8, 12, and 24.

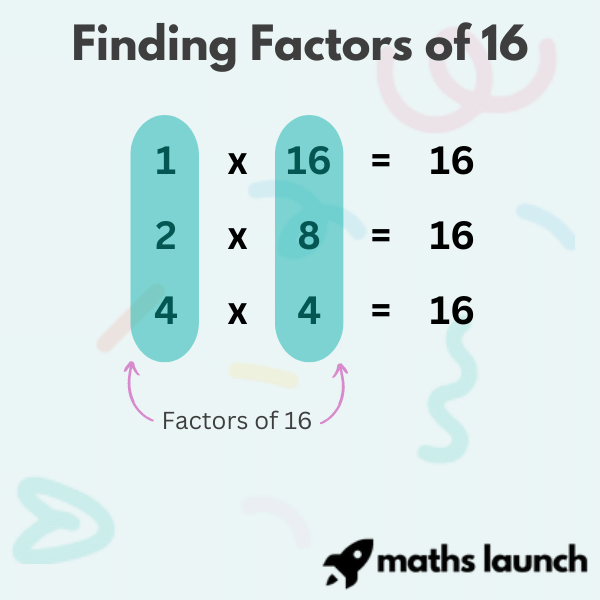
Example 2: Finding Factors of 16

Step 1: Start with the number 16.

Step 2: Divide 16 by 1. The result is 16, so 1 is a factor.

Step 3: Divide 16 by 2. Since the result is 8, 2 is a factor.

Step 4: Divide 16 by 3, 4, 5, 6, and 7. None of these numbers divide 16 evenly.

Step 5: Divide 16 by 8. The result is 2, so 8 is a factor.

Step 6: We can stop here since we have tried all possible numbers. The factors of 16 are 1, 2, 4, 8, and 16.

By following the step-by-step process and applying the divisibility rules when applicable, we can find the factors of any number. It’s like unraveling the secret code of numbers, discovering their smaller components that fit together perfectly.

Now, it’s your turn to practice finding factors on your own. Pick different numbers and see if you can find all their factors. Remember to use the divisibility rules to make the process quicker and more enjoyable.

In the next section, we’ll explore some interesting concepts related to factors, such as common factors and prime numbers.

Common Factors and Prime Numbers

Now that we’ve become experts at finding factors, let’s dive deeper into two interesting concepts: common factors and prime numbers.

Common Factors

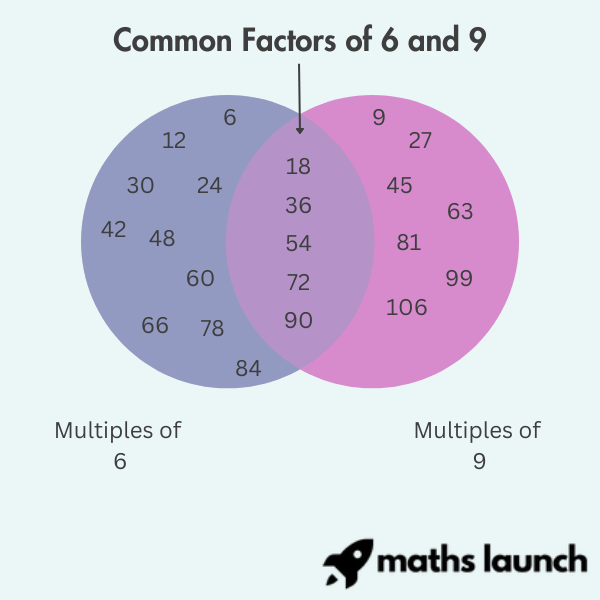
Common factors are the factors that two or more numbers have in common. For example, let’s consider the numbers 12 and 20. The factors of 12 are 1, 2, 3, 4, 6, and 12, while the factors of 20 are 1, 2, 4, 5, 10, and 20. The common factors of 12 and 20 are 1, 2, and 4 since these numbers divide both 12 and 20 without remainders. Common factors help us find the shared divisors of multiple numbers and are useful in various mathematical situations.

Common Factors of Six and Nine

Prime Numbers

Image by Caroline Kulczycky

Prime numbers are unique and fascinating! A prime number is a number that has exactly two factors: 1 and itself.

In other words, a prime number cannot be divided evenly by any other number except 1 and itself. For example, the number 7 is prime because its only factors are 1 and 7. Similarly, the number 11 is also prime because its only factors are 1 and 11. On the other hand, the number 12 is not prime because it has multiple factors (1, 2, 3, 4, 6, and 12).Prime numbers are like the building blocks of the number system, and they have special properties and patterns. They play a crucial role in many areas of mathematics, including cryptography, number theory, and algorithms.

Here are the first few prime numbers: 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, and so on. As we move forward in the number sequence, prime numbers become less frequent and more challenging to identify.

Understanding common factors and prime numbers can help us solve mathematical problems more efficiently. They allow us to simplify fractions, find common denominators, and determine if a number is prime or composite.

Fun Facts About Factors

Factors are not only useful in mathematics, but they also hide some intriguing and fun facts. Let’s uncover a few fascinating tidbits about factors:

Every number has at least two factors: 1 and itself. No matter how big or small a number is, it will always have these two factors.
Perfect Numbers: Some numbers have a special property where the sum of their factors (excluding the number itself) equals the number itself. For example, the number 6 has factors 1, 2, and 3. If we add these factors together (1 + 2 + 3), the sum is 6. Such numbers are called perfect numbers. The first few perfect numbers are 6, 28, 496, and 8128.
Prime Factorization: Every composite number (a number with more than two factors) can be expressed as a product of prime numbers. This representation is called prime factorization. It helps us break down a number into its prime factors and is useful in simplifying fractions and solving equations.
Factors in Nature: Factors can be found in nature as well. For example, the number of petals on a flower often follows patterns determined by factors. Daisies usually have 34, 55, or 89 petals, which are all Fibonacci numbers. Other flowers may have different numbers of petals, but they often reflect factors found in nature.
Multiples and Factors: Multiples and factors are closely related. If a number is a multiple of another number, then the second number is a factor of the first. For example, 12 is a multiple of 3 because it can be evenly divided by 3. Therefore, 3 is a factor of 12.
Factors and Divisibility: Factors help us determine the divisibility of numbers. If a number is divisible by another number without leaving a remainder, then the second number is a factor of the first. Divisibility rules, as we explored earlier, make it easier to identify factors quickly.

Factors are like puzzle pieces that fit together to create the number universe. They have interesting properties and applications that extend beyond mathematics.

By understanding these fun facts about factors, you’ll develop a deeper appreciation for the intricate patterns and relationships present in the world of numbers.

And That’s a Wrap

Let’s recap what we’ve learned in this article:

Factors are the numbers that can divide evenly into a given number without leaving any remainders.
To find factors, we start with the number itself and divide it by smaller numbers, noting the ones that divide evenly.
Divisibility rules, such as those for 2, 3, 4, 5, 6, and 9, can help us quickly determine if a number is divisible by another number without performing the division.
Common factors are the factors that two or more numbers have in common. They help us find shared divisors and are useful in various mathematical situations.
Prime numbers are numbers that have exactly two factors: 1 and themselves. They are the building blocks of the number system and have unique properties and patterns.
Factors have practical applications, such as simplifying fractions, finding common denominators, and determining if a number is prime or composite.
Factors can be found in nature and play a role in patterns observed in the natural world.
Perfect numbers have the special property where the sum of their factors (excluding the number itself) equals the number itself.
Prime factorization allows us to break down a composite number into its prime factors, providing a unique representation.

By understanding factors and their properties, you have gained a valuable tool for solving mathematical problems, exploring patterns, and deepening your understanding of numbers.

Remember, factors are like keys that unlock the secrets within numbers. They allow us to explore their composition, simplify complex concepts, and make sense of the world around us.

So, keep exploring, keep practicing, and keep having fun with factors!